The Northern Lights
Summary
In this activity students will use a circular coordinate grid to plot zones of auroral activity.
Materials
Attachments
Websites
- Aurora Borealis - Northern Lights
Information on the folklore and origins of these cools celestial lights. Includes a video of the phenomenon. - Auroras: Paintings in the Sky
Learn all about the Northern Lights, what causes them, and where they can be found in this site from the Exploratorium. - Iceland Worldwide - Northern Lights
Click on these amazing pictures of the aurora borealis and read explanations of them in the captions. - NORDLYS - Northern Lights
This amazing resource has information on the science behind the aurora borealis, explanations for the lights throughout history, descriptions of different patterns, and lots more, including some amazing photos. - Northern Lights Introduction
Facts, pictures, and a movie of the Northern Lights. - The Aurora Page
Pictures of the Northern Lights taken from space.
For teacher:
- Photos of the Northern Lights
For each student:
- Angle ruler (or protractor)
- Colored pencils
- Where to Find An Aurora worksheet
- Southern Lights worksheet
- Atlas
Background for Teachers
Attachments
Auroras are the beautiful curtains of colored light that are commonly seen in the Arctic and Antarctic regions of Earth. They have a long history of sightings by humans for over 3,000 years. Like lightening and earthquakes, the auroras are natural events.
Auroral light is created by interactions between the sun and Earth. The sun is a mass of electrically charged particles (in the form of a gas). The sun is so hot that it's outer layers blow away in the form of solar wind. It takes an average of three days for this wind to reach Earth. In general, Earth's protective atmosphere and magnetic field protect the planet from this solar wind. Instead of penetrating our atmosphere, particles from the sun collect around Earth and gather in a cavity called the magnetosphere.
 Energized electrons from the sun collide with oxygen and nitrogen in
Earth's atmosphere, producing colorful arrays in Earth's magnetosphere.
The different colors of the auroras are created depending on the
molecules and altitude of collision. A yellow-green color is the result of
an oxygen collision at 100 km. Red auroras occurs at 300 km. Blue light
results from ionized nitrogen molecules, and a purplish-red color is the
result of neutralized nitrogen molecules.
Energized electrons from the sun collide with oxygen and nitrogen in
Earth's atmosphere, producing colorful arrays in Earth's magnetosphere.
The different colors of the auroras are created depending on the
molecules and altitude of collision. A yellow-green color is the result of
an oxygen collision at 100 km. Red auroras occurs at 300 km. Blue light
results from ionized nitrogen molecules, and a purplish-red color is the
result of neutralized nitrogen molecules.
It is easy to graph the auroral zone using angles and a geographic circular grid of the Northern Hemisphere. This activity is a great follow-up to "Tomb Robbers." Prior to this activity, students should be familiar with benchmark angles and the circular grid system. They should be able to plot points on a circular grid and estimate and draw angles with minimal error.
In this activity, students use a circular coordinate grid to plot zones of auroral activity.
 This grid system is different than a
coordinate grid because it is circular.
Astronomers often use circular grids to
identify objects in the night sky. To locate points on a circular grid, start at
the vertex and then move out to the
latitude given by the first coordinate of
an ordered pair, then move
counterclockwise along that circle the number of degrees indicated by the
second coordinate.
This grid system is different than a
coordinate grid because it is circular.
Astronomers often use circular grids to
identify objects in the night sky. To locate points on a circular grid, start at
the vertex and then move out to the
latitude given by the first coordinate of
an ordered pair, then move
counterclockwise along that circle the number of degrees indicated by the
second coordinate.
Vocabulary terms used in this lesson:
angle - The opening between two straight lines that meet at a vertex, measured in degrees.
coordinate grid - A two-dimensional system in which the coordinates of a point are its distances from two intersecting, straight lines called axes.
coordinates - An ordered pair of numbers that identify a point on a coordinate plane or grid.
latitude - A geographic coordinate measured from the equator with positive values going north and negative values going south.
longitude - A geographic coordinate measured from the Prime Meridian (0˚ longitude) with positive values going east and negative values going west.
Intended Learning Outcomes
2. Become mathematical problem solvers.
Instructional Procedures
Attachments
Invitation to Learn
Display several photos of the Northern Lights for the students.
Use some of the following questions to guide a brief class
discussion:
- Do you recognize anything in these photos?
- Has anyone ever seen the Northern Lights?
- Where would you go to see the Northern Lights?
- How are these colors created?
- What is the connection between the Northern Lights and mathematics?
Instructional Procedures
- Have students work alone or in pairs for this activity.
- Distribute a Where to Find an Aurora worksheet to each student.
- Have students label the latitude lines as follows:
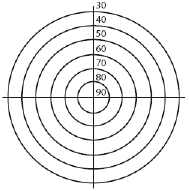
- Next have students estimate and label the unmarked longitude
lines.

- Have students plot the points onto the geographic circular grid for
the outer ring. The points are identified as ordered pairs
(longitude, latitude).
Note: If students are doing this activity after completing "Tomb Robbers," you will want to point out that 0˚ begins at the bottom of the coordinate grid and angles move from this point in a counterclockwise direction. - Have students connect the points in the outer ring, then plot the points in the inner ring.
- Using the scale 1 cm = 1,400 km, have students measure the approximate distance (width) of the ring. To have students find the range of widths, help them take measurements of both, the shortest and longest distances between the inner and outer rings.
- Have students color in the ring with their favorite auroral colors.
- Using an atlas and the student's Where to Find An Aurora
worksheet, hold a class discussion on the following questions:
- Where would you travel in North America to see an aurora?
- Where is the center of the auroral oval located?
- How far is the center from the North Pole?
- What is the range in widths of the auroral oval (in kilometers)?
- If you were located at (205˚, 65˚), where would you look in the sky to see an aurora?
- If you were located at (290˚, 60˚), where would you look in the sky to see an aurora?
Extensions
- Go to the following Web site to study Earth's magnetic field with
a soda bottle magnetometer:
http://image.gsfc.nasa.gov/poetry/workbook/magnet.html - Research the possibility of auroras on other planets/moons. Do auroras only occur where there is an atmosphere?
Assessment Plan
Have students complete the Southern Lights worksheet.
Bibliography
Research Basis
Joram et. al., (2005). Children's Use of the Reference Point Strategy for Measurement Estimation. Journal for Research in Mathematics Education, 36(1), 4-23.
"Mathematics educators frequently recommend that students use strategies for measurement estimation, such as the reference point or benchmark strategy… Relative to students who did not use a reference point, students who used a reference point had more accurate representations of standard units and estimates of length."
Updated: 02/05/2018


 UTAH EDUCATION NETWORK
UTAH EDUCATION NETWORK

 Justin
Justin Braxton
Braxton Dani
Dani Kayla
Kayla Katie
Katie Matthew
Matthew Rob
Rob Val
Val
